Average peak values
The example consists of a simple plate imposed on four supports in internal nodes. These supports represent columns, for example.
This plate is calculated linearly with its own weight as the only load case.
The results for the reinforcement moment at the top mx, D+ show the expected peak values at the supports.

The peak values can be reduced in different ways.
At first the supports are made flexible.

Subsequently, the results can be further decreased by means of averaging strips.
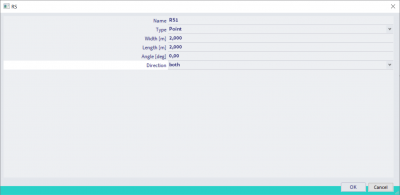
We choose an averaging strip around with a length and width of 2m. The direction in which the averaging is done, is set on both directions.
You can find an elaborated explanation about how to use the averaging strips in chapter 5.6 of our basic manual.
To indicate that the averaging strip is taken into account for the representation of the results, you also have to tick on the "averaging of peak" in the properties window of the results.
The result looks like this:
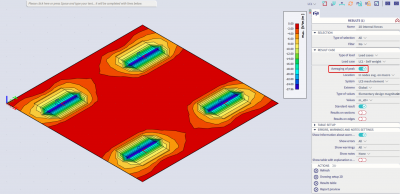
The averaging strips help to average the peak values, but if you still don't find the result accurate enough you can model the supports as surface supports instead of point supports.
To do this, subregions must be created in the plate at the position of the columns. A surfacesupport with a subsoil is then assigned to this subregion. You can assign the appropriate stiffness properties to the subsoil, calculated from the stiffness of the column. Finally, the point supports must be removed.
In this example, we have applied this to one of the four supports to demonstrate the difference.
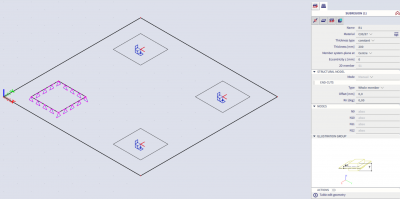
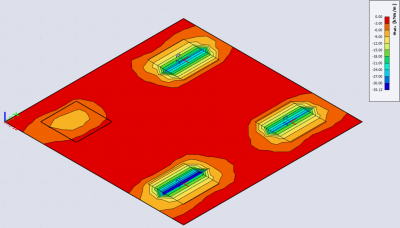
Notice that the peak values no longer occur.
Working with a flexible subsoil instead of a point support results in a model that is closer to reality. The occurrence of peak values is also greatly reduced as a result.